A major thrust in our group involves the development of novel field theoretic simulations (FTS) techniques for the study of systems that can only be crudely modeled by existing methods. FTS are a broad class of numerical approaches that include self-consistent field theory (SCFT), which is a ubiquitous method for studying polymer melts, blends, solutions, and block copolymers. This family of methods is a highly coarse-grained description of a system where polymers are typically modeled as Gaussian chains with purely local interactions. After writing down the partition function for such a model, one uses a particle-to-field transformation to exactly transform the model to a statistical field theory that can be evalulated numerically on a collocation grid or attacked with a variety of analytical techniques. The advantage of the FTS framework is that one can frequently resolve the model using significantly fewer collocation points in the field theory than there are particles in the underlying model, leading to more efficient simulations.

A significant shortcoming of many current implementations of polymer field theory is that they completely miss correlations due to excluded volume, which makes this approach invalid for systems where correlations become important. This includes capturing the packing of monomers near a hard wall, or capturing the manner in which nanoparticles would affect the conformations available to a polymer chain. Correlations are missed because the monomer densities are typically described as point particles (mathematically, the density is defined as a sum of delta functions, \(\hat{\rho}(r) = \sum_i \delta(r-r_i)\)), and the interactions are purely local. A pair potential would be of the form \(u(r) = \chi \delta(r)\), so monomers would only interact when they are precisely on top of each other. This is clearly inadequate if one wishes to include objects such as nanoparticles that are significantly larger than a typical monomer size.

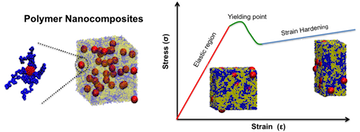
Our approach has been to show that by simply using a “smearing” function that describes the shape of the monomer, we can move beyond the point-particle approximation to include objects of a finite size (e.g., nanoparticles) in our theories. We simply replace our microscopic density function with a sum over these smearing functions, \(\hat{\rho}(r) = \sum_i \Gamma(r-r_i)\) where \(\Gamma(r)\) describes the shape of the object, as shown in Koski et al., 2013. We call this framework polymer nanocomposite field theory (PNC-FT); it is a generalization of the prior hybrid particle/field theory pioneered by Sides et al., and our approach enables efficient study of the fully fluctuating field theory using the complex Langevin technique.

There are few limitations on the choice of the smearing function, which enables us to study a wide range of polymer nanocomposite systems. We typically use a Gaussian distribution to describe the distribution of mass within a coarse-grained polymer monomer and spherical step functions with a soft interface to describe the shape of spherical nanoparticles. It is also possible to employ anisotropic smearing functions, enabling us to study rod- and plate-like nanoparticles. We have also further extended the theory to describe polymer-grafted nanoparticles with arbitrary grafting architecture such as homopolymer grafting, grafting with mixtures of polymers, block copolymers, and Janus grafting, which has allowed us to study the interfacial activity of different designs of grafted nanoparticles and their effect on the interfacial tension in polymer (see Koski et al., 2015) blends.

One of our major methodological developments has been our ability to translate our PNC-FT models to the recently-proposed dynamic mean-field theory (DMFT) outlined by Fredrickson and Orland, which enables us to study the same family of models using a dynamic theory that can qualitatively capture the effects of processing on the structure of inhomogeneous polymer nanocomposites. The framework is qualitatively similar to the single-chain in mean-field (SCMF) approach of Mueller et al. and the self-consistent Brownian dynamics (SCBD) method of Ganesan et al., but the solid theoretical basis provided by Fredrickson and Orland provides the possibility for systematically improving the methods by adding hydrodynamic interactions, for example.

We have employed our dynamic mean field theory (DMFT) to study how solvent annealing affects the distribution of nanoparticles in block copolymer thin films (see image above). Solvent annealing is a process through which a block copolymer thin film is exposed to the vapor of a volatile solvent, which absorbs into the film, swelling and plasticizing the block copolymer. Solvent vapor annealing is widely used to reduce the density of defects in block copolymer films, and it is finding increased usage in block copolymer nanocomposite thin films. We have shown that for lamellar films swollen with a neutral solvent, if we tune the interfacial activity of the nanoparticles by varying the grafting density of polymers on the particle surface, we can tune the equilibrium distribution of nanoparticles. At some particular surface chemistries, we find that the solvent will displace the particles from the free surface, leading to interfacially-active particles becoming mixed in the polymer film. Under these conditions, when the solvent is rapidly evaporated from the film, we can trap the particles in the distribution they adopt in the swollen state. However, if the solvent is removed slowly, then the particles have sufficient time to anneal back to their equilibrium distribution in the dry state. This work was recently published in Soft Matter.

Amorphous solids are ubiquitous in our daily lives, for example as polymer glasses in lenses and protective coatings or as thin organic glass films as active layers in electronic devices. Despite their wide-spread use, the basic physics of glass formation remain poorly understood. One common theme in many of the theories of glass formation, particularly the entropy theories that can be traced back to the original Adam-Gibbs theory, is that the dynamic slowdown that leads to the glass transition is accompanied by a growing dynamical length scale. The essential idea is that as the temperature is lowered, the dynamics become increasing cooperative; if more polymer segments must move together for relaxation to occur, this requires overcoming a larger energy barrier, which in turn leads to slower dynamics. This basic view has lead to many years of research studying the dynamics of glasses (primarily polymers) under nanoscale confinement. Our first independent contribution in this area was to show that confinement effects cannot be universally explained by this growing length scale. We designed a family of glass-forming polymers that have similar extents of cooperative dynamics. Upon confinement to planar thin films, we found that the confinement effects were drastically different due to differences in the polymer structure near the interface, which strongly suggests that the extent of cooperative dynamics cannot uniquely explain the confinement effects. We later showed that for flexible polymers that do not order near the interface, this dynamic length scale may be relevant, though we were of course limited to the time scales easily accessible by molecular dynamics.

Entangled polymers are increasingly used in highly confined geometries, and emerging applications include the formation of nanocomposites at exceptionally high nanoparticle concentrations (see the CaRI simulations in the image above) and as rheological modifiers in fracking fluids that must flow to nanoscopic pores. When the confining dimension becomes comparable to the chain dimensions, one should anticipate that the chains become deformed, potentially affecting their ability to entangle with each other. In collaboration with Prof. Karen Winey (Penn, Materials Science and Engineering), we have been investigating how nanoscale confinement affects the entanglement density and dynamics of polymers in the melt state (see Tung et al. and Sussman et al.). Our simulations showed that when the chains were confined to length scales below their bulk end-to-end distance, the entanglement density is reduced, and this effect is significantly more pronounced for two-dimensional confinement to nanopores. The reduction in entanglement density is reflected in a substantial increase in the diffusivity of the polymers, and we are currently working to quantitatively compare the simulations to experiments that measure chain conformation and diffusivity.

The results of this prior work on the dynamics of amorphous and entangled polymers under nanoscale confinement will be used as a fundamental framework for our current work studying the dynamics of polymers during capillary rise infiltration (CaRI) into a dense nanoparticle packing. This method for forming polymer nanocomposites developed in the Lee lab (Penn, Chemical and Biomolecular Engineering) enables the scalable production of polymer nanocomposites with nanoparticle loadings above 50\(%_{vol}_\). The pores in this nanoparticle packing are on the order of the chain dimensions, so it is unclear whether bulk dynamic properties will govern the infiltration process or the extent to which confinement effects will play a role. In our first simulations of this process, we compared the infiltration dynamics of two unentangled polymer chain lengths with a characteristic size below the pore sizes and one comparable to the pore sizes. For the longer chain length, we found that polymers frequently moved through the packing in a stretch-and-hop motion to move through the tighter constrictions within the nanoparticle packing.

A wide range of soft amorphous solids, such as coarsening foams and emulsions, pastes, and even living cells, have anomalous rheological properties where the modulus exhibits a weak power-law behavior, \(G^\ast (\omega) \sim \omega^{\beta}\) with \(\beta\) a non-trivial exponent typically in the range \(0.1 < \beta < 0.3\). While phenomenological models have been developed to describe this class of behavior known as soft glassy rheology, there had not been any particle-level models that exhibit this weak power law behavior. To that end, along with John Crocker (Penn, Chemical and Biomolecular Engineering), we have developed a novel model where soft glassy rheology is emergent. Our model consists of a polydisperse collection of soft spheres (bubbles) that represent a coarsening foam. Gas flows from the small bubbles to the large bubbles driven by Laplace pressure differences between the bubbles, and our simulations proceed by following a quasi-static ripening process. We allow a small amount of ripening, subsequently quench the system to an energy minimum, and repeat. Interestingly, we find that the dynamics of the bubbles is super diffusive at long times where \(\left< r^2 \right> \sim t^{\alpha}\), with \(\alpha = 1.37\). By analyzing the stress fluctuations and using analysis from the microrheology community, we are able to connect these dynamics to the SGR power law rheology behavior to find that our model predicts \(\beta = 0.17\). To understand the origin of this behavior, we tracked the dynamics of the system along its 3N-dimensional energy landscape, and we found that the trajectories have the properties of a self-similar fractal and the dimensionality of the fractal can be directly related to the power law exponent \(\beta\). Our work provides a deep connection between the dynamics of these systems on a gradually evolving energy landscape and their rheological properties.

Many nanotechnology applications, such as semiconductor manufacturing, require the production of stable structures of glass-forming materials (molecular glasses, polymers) with features on the order of 5-30 nm in size. While creating such structures is a tremendous challenge by itself, the problem is exacerbated because the properties of glass-formers can change dramatically when they are confined to such small length scales. Their glass transition temperature and elastic constants can change from their bulk values in counter-intuitive ways. Fortunately, these length scales where confinement has an effect are reasonable to simulate directly with molecular dynamics and Monte Carlo simulations. We explore how a variety of glass-forming materials such as molecular glasses and polymer glasses are affected by nanoscale confinement using these simulation techniques in an effort to control and understand the origins of the property changes.

Experiments over the past decade have clearly demonstrated that the addition of a small amount of nanoparticles to a polymer matrix can have a tremendous impact on their mechanical properties. Often times, however, the nanoparticles must be decorated with polymer brushes in order to prevent them from aggregating in the polymer matrix, which can degrade the properties of the material. These polymer brushes can be the same polymer as the host matrix, and the dispersion of the nanoparticles and the property changes they impart can depend strongly on the relative molecular weights of the polymer chains as well as the interactions between the polymer brushes, nanoparticles, and the matrix polymer. We are using a series of coarse-grained models to understand how brush-decorated nanoparticles affect the non-linear mechanical properties of glassy polymers.